Determine operating mode
Determine relationship between time and speed.

Linear

Uniform Motion		$$v=\frac{s}{t}$$
Constant Acceleration/Deceleration		$$s=v_0 \cdot t_1+\frac{1}{2} \cdot v_{max} \cdot t_1 $$ $$s=v_0 \cdot t_1+\frac{1}{2} \cdot v_{max} \cdot t_1 $$ $$=v_0 \cdot t_1+ \frac{1}{2} \cdot a \cdot {t_1}^2$$
Positioning		$$v_{max}=a \cdot t_1$$ $$s=v_{max} \cdot t_1+v_{max} \cdot t_2$$ $$=a \cdot {t_1}^2+v_{max} \cdot t_2$$

Rotary

Uniform Motion		$$1 rad=\frac{360°}{2\pi}$$ $$\omega=\frac{2πn}{60}$$
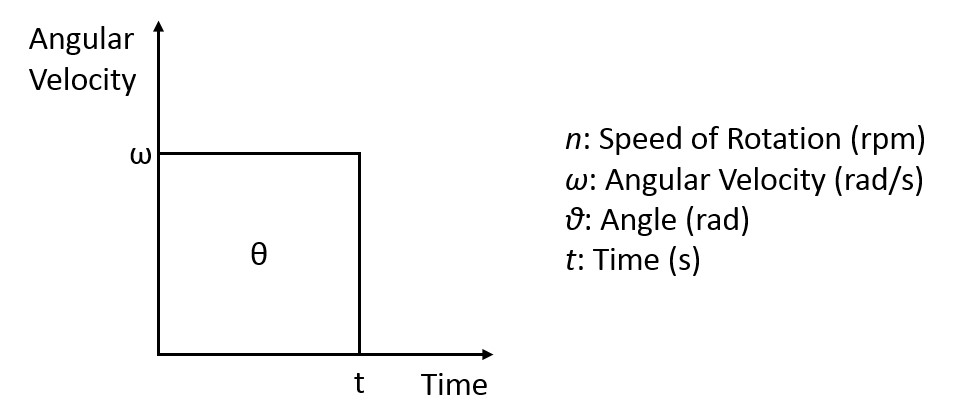
Uniform Motion		$$ω=\frac{θ}{t}$$ $$n=\frac{30}{\pi} \cdot \frac{θ}{t}$$
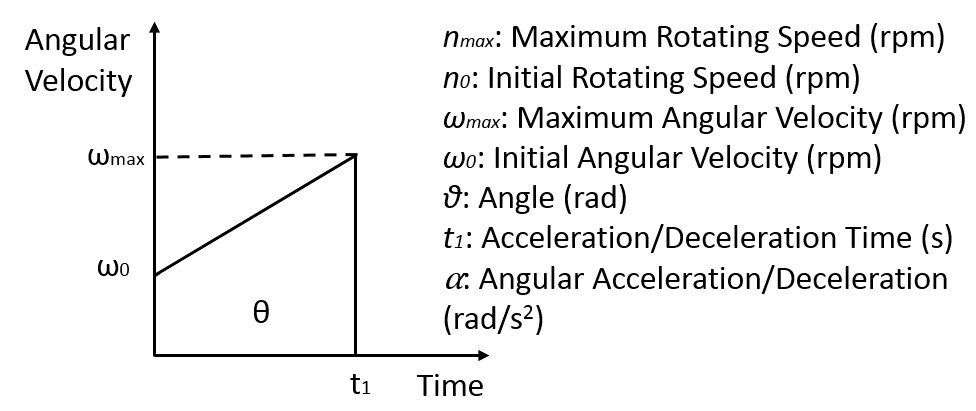
Constant Acceleration/Deceleration		$$ω_{max}=ω_0+α \cdot t_1$$ $$n_{max}=n_0+\frac{30}{\pi} \cdot α \cdot t_1$$ $$θ=ω_0 \cdot t_1+\frac{1}{2} \cdot ω_{max} \cdot t_1$$ $$=ω_0 \cdot t_1+\frac{1}{2} \cdot α \cdot t_1$$ $$=\frac{30}{\pi} \cdot n_0 \cdot t_1+\frac{1}{2} \cdot α \cdot {t_1}^2$$
Positioning		$$ω_{max}=α \cdot t_1$$ $$n_{max}=\frac{30}{\pi} \cdot α \cdot t_1$$ $$θ=ω_{max} \cdot t_1+ω_{max} \cdot t_2$$ $$=α \cdot {t_1}^2+ω_{max} \cdot t_2$$ $$=α \cdot {t_1}^2+\frac{30}{\pi} \cdot n_{max} \cdot t_2$$