|
Point
|
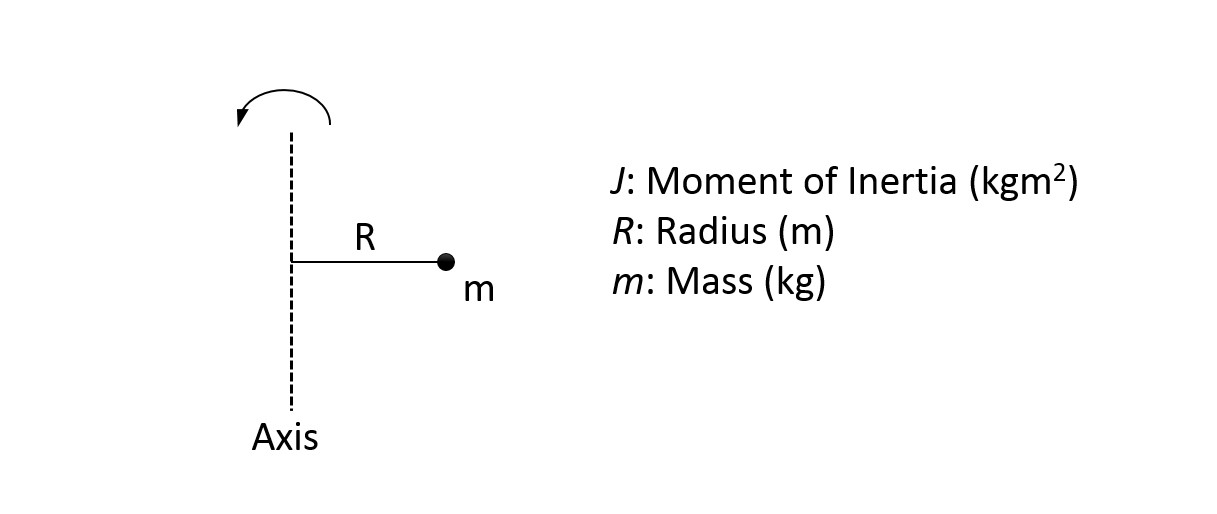
|
$$J=m \cdot R^2$$
|
|
Solid Sphere
|

|
$$J=\frac{2}{5} \cdot m \cdot R^2$$
|
|
Hollow Sphere
|
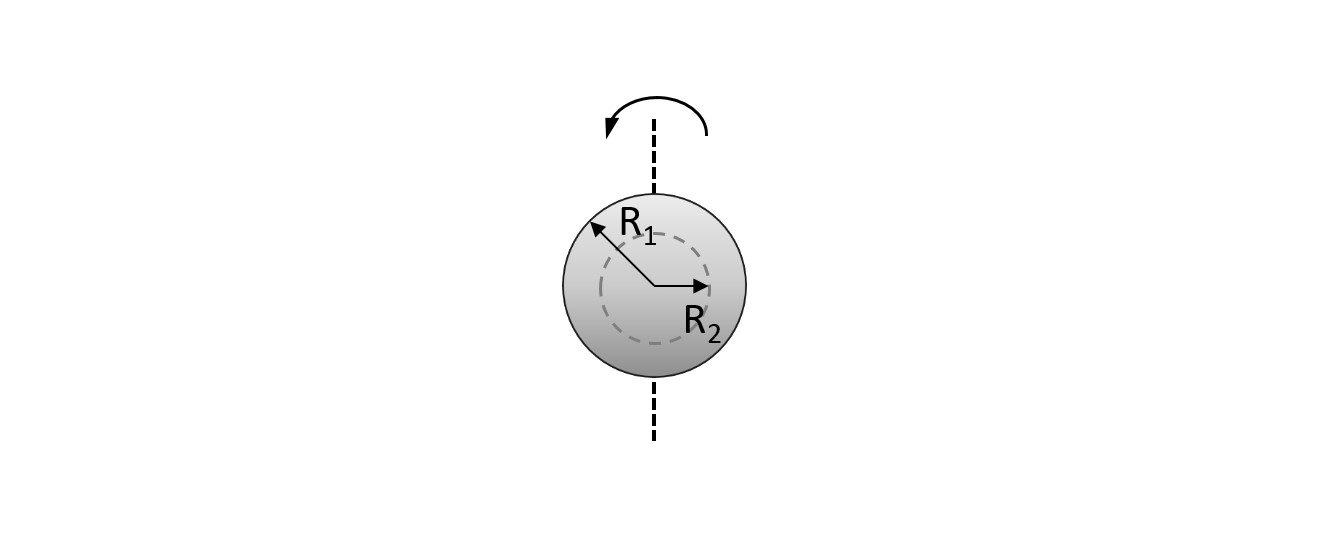
|
$$J=\frac{2}{5} \cdot m \cdot \left(\frac{{R_1}^5-{R_2}^5}{{R_1}^3-{R_2}^3}\right)$$
Hollow thin-walled sphere:
$$J=\frac{2}{3} \cdot m \cdot {R_1}^2$$
|
|
Solid Cylinder
|
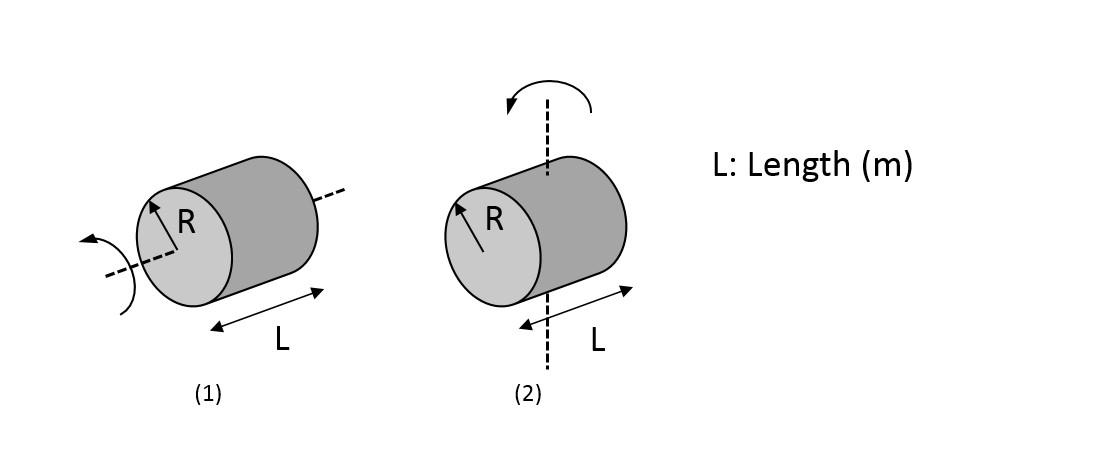
|
(1)$$J=m \cdot R^2$$
(2)$$J=\frac{1}{4} \cdot m \cdot R^2+\frac{1}{12} \cdot m \cdot L^2$$
|
|
Eccentric Solid Cylinder
|

|
$$J=J_c+m \cdot {R_e}^2$$
|
|
Hollow Cylinder
|
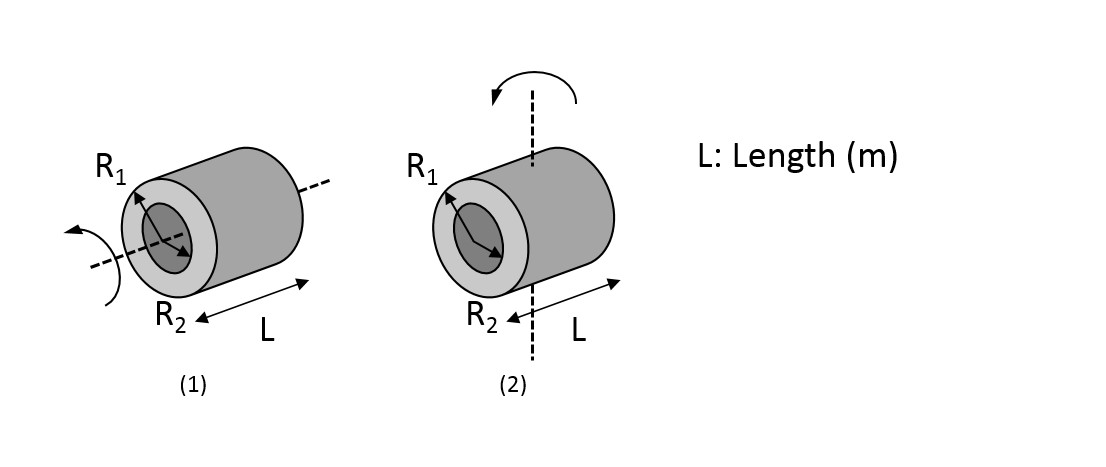
|
(1)$$J=\frac{1}{2} \cdot m \cdot ({R_1}^2+{R_2}^2)$$
Hollow thin-walled cylinder:
$$J=m \cdot {R_1}^2$$
(2)$$J=\frac{1}{4} \cdot m \cdot ({R_1}^2+{R_2}^2+\frac{L^2}{3})$$
Hollow thin-walled cylinder:
$$J=\frac{1}{2} \cdot m \cdot {R_1}^2+\frac{1}{12} \cdot m \cdot L^2$$
|
|
Circular Cone
|
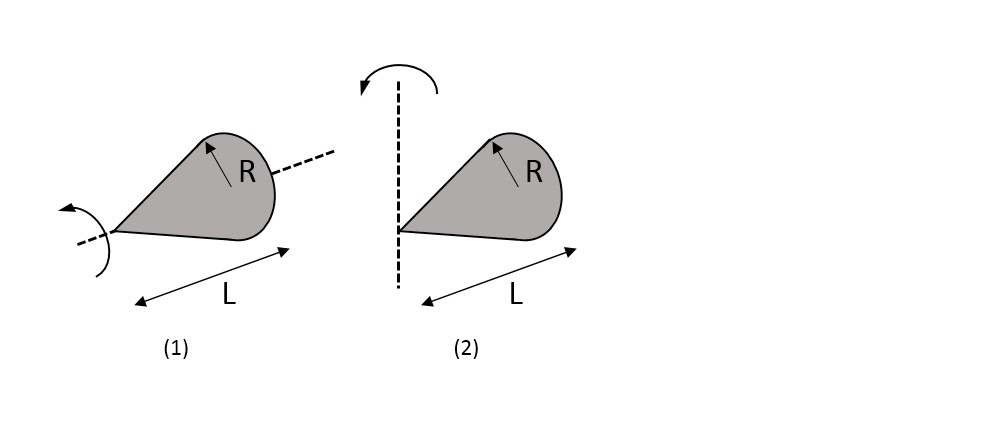
|
(1)$$J=\frac{3}{10} \cdot m \cdot R^2$$
Hollow thin-walled cone:
$$J=\frac{1}{2} \cdot m \cdot R^2$$
(2)$$J=\frac{3}{20} \cdot m \cdot (R^2+4L^2)$$
Hollow thin-walled cone:
$$J=\frac{1}{4} \cdot m \cdot (R^2+2L^2 )$$
|
|
Cuboid
|
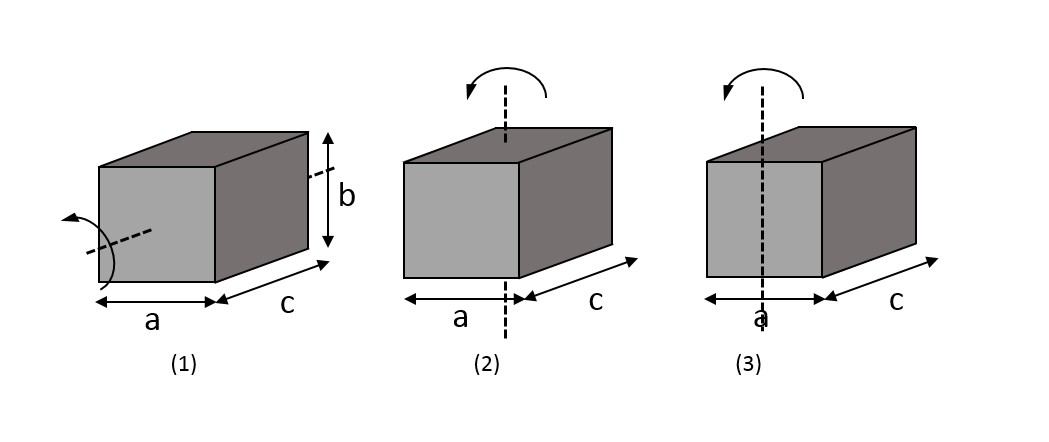
|
(1)$$J=\frac{1}{12} \cdot m \cdot (a^2+b^2 )$$
(2)$$J=\frac{1}{12} \cdot m \cdot (a^2+c^2 )$$
(2)$$J=\frac{1}{12} \cdot m \cdot (a^2+4c^2 )$$
|
|
Rod
|
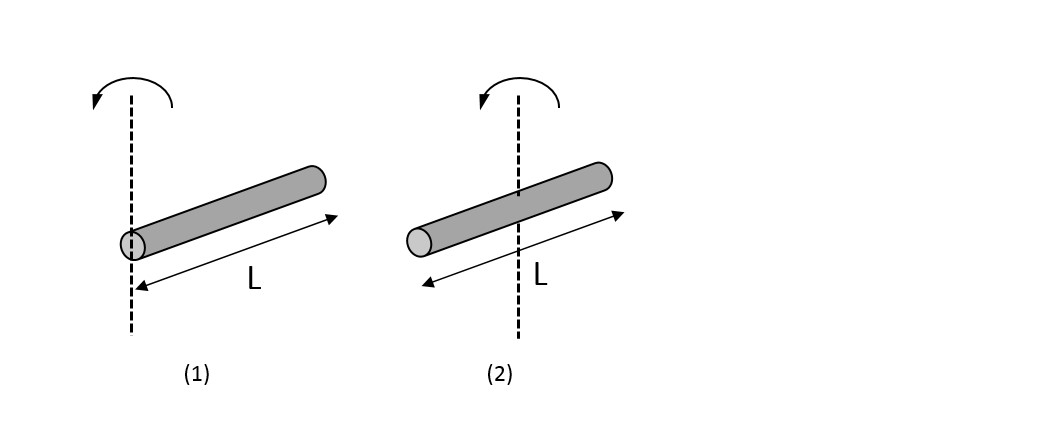
|
(1)$$J=\frac{1}{3} \cdot m \cdot L^2$$
(2)$$J=\frac{1}{12} \cdot m \cdot L^2$$
|